![∑ ∑
||X - g g⋆||2 = [X - g g⋆][X ⋆ - g⋆g ]
i,j ij i j i,j ij i j ij i j
i⁄=j i⁄=j
∑ [( R I) ( R I)( R I)]
= X ij + ιXij - gi + ιgi gj - ιgj
i,i⁄=jj
[( R I) ( R I)( R I) ]
∑ X ij - ιXij - gi - ιgi gj + ιgj
= [(XR - gRgR - gIgI) + ι(XI + gRgI - gIgR )]
i,j ij i j i j ij i j i j
i⁄=j
[(XR - gRgR - gIgI) - ι(XI + gRgI - gIgR )]
∑ ij i j i j ij i j i j
= S0S ⋆0
i,j
i⁄=j](antsol18x.png)
gis are complex functions. One can therefore write S in terms of giI and g iR, the real and imaginary parts of gi and minimize with respect to giI and g iR separately. It is shown here that the complex arithmetic achieves exactly this and the results are same as that given by complex calculus. The superscripts I and R in the following are used to represent the real and imaginary parts of complex quantities.
Expanding Eq. 5, ignoring wijs and writing it in terms of real and imaginary parts we get
![∑ ∑
||X - g g⋆||2 = [X - g g⋆][X ⋆ - g⋆g ]
i,j ij i j i,j ij i j ij i j
i⁄=j i⁄=j
∑ [( R I) ( R I)( R I)]
= X ij + ιXij - gi + ιgi gj - ιgj
i,i⁄=jj
[( R I) ( R I)( R I) ]
∑ X ij - ιXij - gi - ιgi gj + ιgj
= [(XR - gRgR - gIgI) + ι(XI + gRgI - gIgR )]
i,j ij i j i j ij i j i j
i⁄=j
[(XR - gRgR - gIgI) - ι(XI + gRgI - gIgR )]
∑ ij i j i j ij i j i j
= S0S ⋆0
i,j
i⁄=j](antsol18x.png) | (14) |
where
![[ ] [ ]
S0 = XR - gR gR - gIgI + ι XI + gR gI- gIgR
ij i j i j ij i j i j](antsol19x.png) | (15) |
Taking partial derivative of S with respect to giR and reintroducing w ij, we get
![∑
∂S--= { [- gR + ιgI] S⋆ - S [gR + ιgI]}w
∂gRi j j j 0 0 j j ij
j⁄=i
∑ [ ⋆ ⋆]
= - S0gj + g jS0 wij
jj⁄=i
∑
= - 2 Re (S0gjwij)
jj⁄=i
∑ [( ) ( ) ]
= - 2 XRij - gRi gRj - gIigIj gRj + XIij + gRi gIj - gIigRj gIj wij
j
∑j⁄=i[ ]
= - 2 XRijgRj - XIijgIj - gRi gIj2 - gRi gRj 2 wij
j
j⁄=i](antsol20x.png) | (16) |
Therefore,
![-∂S- ∑ [ 2 R ]
∂gR = - 2 Re (Xijgj) - |gj| gi wij
i jj⁄=i](antsol21x.png) | (17) |
Equating ∂S _ ∂giR to zero, we get
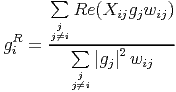 | (18) |
Similarly
![∂S-- ∑ [ 2 I]
∂gIi = - 2 Im (Xijgj) - |gj| gi wij
jj⁄=i](antsol23x.png) | (19) |
Therefore the equivalent imaginary part of Eq. 18 is
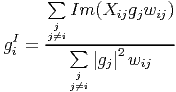 | (20) |
writing gi = giR + ιg iI and substituting for g iR and g iI from Eq. 18 and 20 respectively, we get
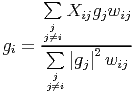 | (21) |
This is same as Eq. 8, which was arrived at by evaluating a complex derivative of Eq. 5 as ∂S∕∂gi⋆, treating g i and gI⋆ as independent variables. Evaluating ∂S ∂gi = 0 would give the complex conjugate of Eq. 21. Hence, ∂S∕∂gi gives no independent information not present in ∂S∕∂gi⋆.