Route-c

Moderately increase the UVRAN to a value of, say $4K\lambda$ (refer to the arguments in Section 2.1.2) but apply no selection on the antennas to be used. The data so calibrated is shown in panel 1 of Fig. 3. Clearly, a number of poorly calibrated baselines are included. Arguably these baselines can be flagged and a better solution found. However, first of all, the data from these baselines is not intrinsically corrupted - it is just wrongly modeled. Secondly, as discussed in Section 3, from the point of view of automation of this process, flagging is not a good (or even correct!) option.

Thus, clearly, Route-b is the most appropriate. Therefore, solution for the gains of the Central square and the nearest arm-antennas W01, W02, E02 and S01 were found using the map obtained using baselines up to $2K\lambda$ (see the panel 3 of Fig. 2). The data so calibrated was imaged and the resulting image was also self-calibrated once. This image was then used as the image model in the next step. If this step was successful, then this image (Fig. 4) should produce a better model for the observed visibilities corrected for the antenna based offsets ( $V_{ij}^{Cor}=V^{obs}_{ij}/g_i g^\star_j$). To check this we Fourier inverted the map to obtain the model visibilities (i.e. $V_{ij}^{model}$). If the model matches the data then $V_{ij}^{Cor}$/ $V_{ij}^{model}$ should be close to unity, which is indeed true (see panel 2 of Fig. 4 for the normalized plot).

Figure: Comparison between Route-b and -c the bootstrapping. Right hand panel i.e. panel 1 and panel 2 show the data calibrated using the gains from Route-c and Route-b respectively.

Figure: Map using UV $_{max}=4K\lambda$ i.e. Central square antenna along with W01,W02,E02 and S01 and the normalized visibilities i.e. $V_{ij}^{Cor}$/ $V_{ij}^{model}$.

Figure: Map using UV $_{max}=6K\lambda$ i.e. E03, S02, S03 and W03 are also added and the normalized plot.

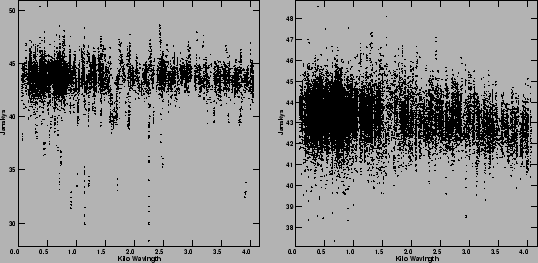
In the next iteration, UVRAN was increased to $6K\lambda$ and antennas E03, S02, S03 and W03 were also included. Input image model was the image from previous iteration (see Fig. 4). (see Fig. 5 for the map and the normalized plot. Map was also Self-calibrated).

Figure: Map using UV $_{max}=8K\lambda$ with all the antennas and the full calibrated data.

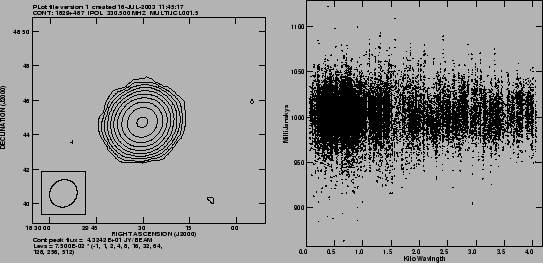
In the final iteration, we also included E04, E05, E06, W04, W05, S04, W06 and S06 (i.e. the entire array). Again, as before, the input model image was the image from the previous iteration (the image in Fig. 6) and UVRAN increased to $8K\lambda$. Obviously, the gains so obtained should be applicable to all the visibilities (i.e. UVRAN=0). Panel 2 in the Fig. 6 shows the calibrated amplitude for the whole data. Again, if the method was successful, then $V_{ij}^{Cor}$/ $V_{ij}^{model}$ should be close to unity. Fig. 7 shows that this is indeed true. Fig. 7 also shows the final image at full GMRT resolution.

Figure: Map using all the data and the normalized plot.