The purpose of this problem is to illustrate the use of the Tully-Fisher relation for determining distances to galaxies, as well as computing mass-to-light ratios.
- (a)
- After plug-and-play, we find
MB = -21.83
- (b)
- The distance modulus is
B - MB = 34.05 = 5 log d - 5
- (c)
- The relation gives
log R25 = 1.4357
- (d)
- The sky brightness (for example in one square arcsecond) is larger
by a factor of
Isky/I25 = 100.4(25-22) = 101.2 = 15.8
- (e)
- Our mass is (remembering to convert km/s to m/s and kpc to m)
M = v2 R / G = 1.32 × 1042 kg = 6.62 × 1011 Msun
- (f)
- The B magnitude of the Sun is
MB,sun = MV,sun + ( B - V ) = 4.83 + 0.64 = 5.47
L / Lsun = 100.4(4.83 - MB) = 1010.66 = 4.61 × 1010
- (g)
- The mass-to-light ratio is
M / L = 6.62 × 1011 Msun / 4.61 × 1010 Lsun = 14.36 Msun / Lsun
We compute the sums (using a handy calculator)
 (di /
(di /
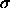 i2)
= 56.1082
i2)
= 56.1082

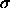 i-2
= 3.5485
i-2
= 3.5485
davg = 56.1082 / 3.5485 = 15.81 Mpc
The uncertainty in our estimate is therefore
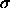 d =
3.5485-1/2 = 0.53 Mpc
d =
3.5485-1/2 = 0.53 Mpc
dVirgo = 15.81 ± 0.53 Mpc
The Hubble constant is given by
H0 = v / d = 1136 km/s / 15.81 Mpc = 71.85 km/s/Mpc.
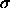 H0
/ H0 =
H0
/ H0 = 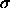 d
/ d = 0.53 / 15.81 = 0.0335
d
/ d = 0.53 / 15.81 = 0.0335
H0 = 71.9 ± 2.4 km/s/Mpc
After correction for 168 km/s infall toward Virgo, the recession velocity is 1304 km/s giving
H0 = 82.5 ± 2.8 km/s/Mpc
The Hubble time is then
1 / H0 = 1/82.5 s Mpc/km × 3.086 × 1019 km / Mpc = 3.74 × 1017 s = 11.85 Gyr
tH = 11.85 ± 0.40 Gyr.
With a velocity dispersion of 666 km/s we get the virial mass of
Mvir = 1.54 × 1045 kg = 7.70 × 1014 Msun
To cross the diameter of the Virgo cluster
D = 3 Mpc × 3.086 × 1019 km/Mpc = 9.26 × 1019 km
tcross = 1.39 × 1017 s = 4.4 Gyr
The Hubble velocity between opposite sides of the cluster is
vH = 3 Mpc × 82.5 km/s/Mpc = 248 km/s
vH /
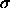 r
= D H0 /
r
= D H0 / 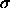 r
= (D/
r
= (D/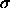 r) /
(1/H0) = tcross / tH
r) /
(1/H0) = tcross / tH
Using T = 7 × 107 K and R = 1.5 Mpc
- (a)
- The luminosity is given by
Lx = ( 4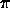 R2 / 3 )
R2 / 3 )
 ff
= 1.5 × 1036 W
ff
= 1.5 × 1036 W
 ff =
1.5 × 1036 W / 4.155 × 1068 m3
= 3.61 × 10-33 W/m3.
ff =
1.5 × 1036 W / 4.155 × 1068 m3
= 3.61 × 10-33 W/m3.
ne = [ 3.61 × 10-33 / 1.42 × 10-40 ]1/2 [ 7 × 107 ]-1/4 = 55.12 m-3
- (b)
- If the IGM gas is hydrogen, and it is fully ionized (which it is) the
hydrogen density will be equal to the electron density, so the mass density
in the IGM will be
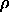 = ne mH
= 9.206 × 10-26 kg m-3
= ne mH
= 9.206 × 10-26 kg m-3
Migm = 9.206 × 10-26 kg m-3 × 4.155 × 1068 m3 = 3.82 × 1043 kg = 1.91 × 1013 Msun.
- (c)
- Combining (b) and the results from problem 3, we get
Migm / Mvir = 1.91 × 1013 Msun/ 7.70 × 1014 Msun = 0.025
- (d)
- The total mass-to-light ratio (using the virial mass) is
Mvir / L_V = 7.70 × 1014 Msun / 1.2 × 10^12 Lsun = 642
Mgal ~ 10 L_V = 1.2 × 1013 Msun
- (e)
- The hot electrons and protons form a gas, with an energy density of
uigm = 3 nigm k T / 2 = 3 ne k T
uigm = 1.60 × 10-13 J m-3
tcool = uigm / ff
= 1.60 × 10-13 J m-3 /
3.61 × 10-33 W m-3 =
4.43 × 1019 s = 1.4 × 1012 yrs
ff
= 1.60 × 10-13 J m-3 /
3.61 × 10-33 W m-3 =
4.43 × 1019 s = 1.4 × 1012 yrs
We calculate the Eddington luminosity
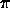 G M c /
G M c /
 = 1.26 × 1031 W ·
( M / Msun )
= 1.26 × 1031 W ·
( M / Msun )
using the electron scattering (Thomson) opacity. In terms of solar luminosities
and thus 108 Msun gives 3.3 × 1012 Lsun ( 1.26 × 1039 W ), which is in the range of quasar luminosities.
Each kilogram of matter brought from "infinity" to a radius of q Schwarzschild radii will give up the change in gravitational energy (which is zero at R of infinity)
and thus delivers an appreciable fraction of its rest-mass energy mc2! For q=3, we get c2/6 per kg, or 1.5 × 1016 J/kg. Thus, to maintain the Eddington luminosity we calcluated above, we need to accrete mass at the rate
or
and thus our black hole just has to munch on a star every year or so (or one big star every few years)!